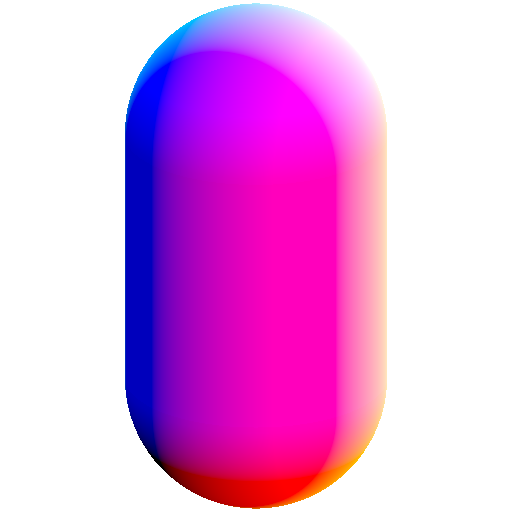
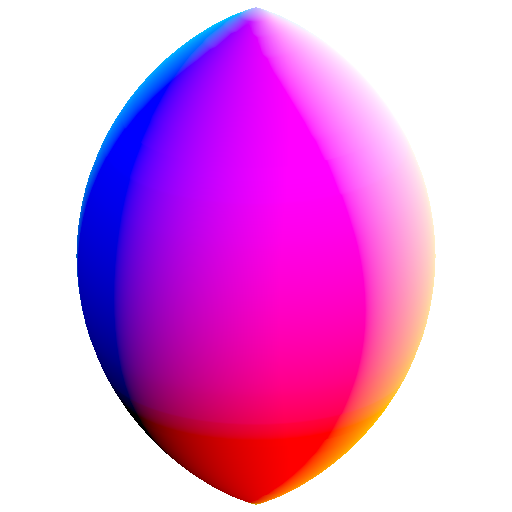
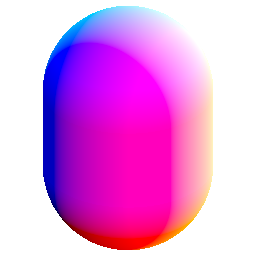
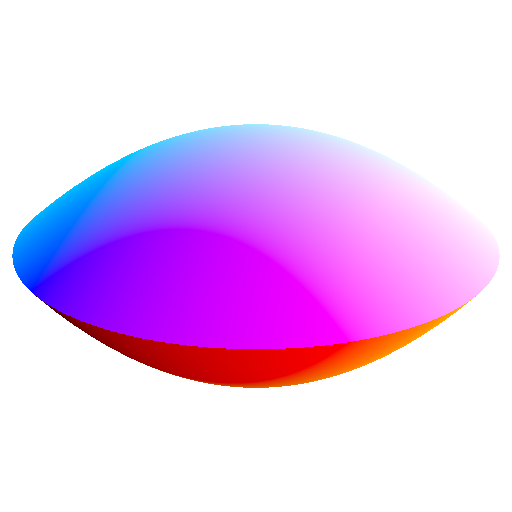
shapes!

im going to add explanations and equations for all this later don't worry

also yes no matter what i do the descriptions like to be to the left of the images instead of underneath them, css is hard

Dual Pairs of 3D Surfaces

capsules/biconics, anticapsules/lemonoids

Image + Name	Description	Symmetry	Dual
Capsule	Surface of revolution of a stadium	D∞	Biconic
Elliptical Bisegmentoid (Biconic)	Two copies of a surface of revoluion of an elliptical segment (non-uniformly scaled circular segment), attached circlewise	D∞	Capsule (a<2r)
Biparaboloid (Biconic)	Two paraboloids attached circlewise	D∞	Capsule (a=2r)
Bihyperboloid (Biconic)	Two hyperboloids attached circlewise	D∞	Capsule (a>2r)
Anticapsule	Like the capsule, it's a surface of revolution of a stadium, but along the other axis Also the convex hull of a torus, conversely making lemonoids the convex core of torets	D∞	Lemonoid
Elliptical Segmentoidal Lemon (Lemonoid)	A surface of revoluion of an elliptical segment	D∞	Anticapsule (a<2r)
Paraboloidal Lemon (Lemonoid)	Surface of revolution of a parabola, other axis compared to a paraboloid	D∞	Anticapsule (a=2r)
Hyperbolic Lemon (Lemonoid)	Surface of revolution of a hyperbolic segment	D∞	Anticapsule (a>2r)

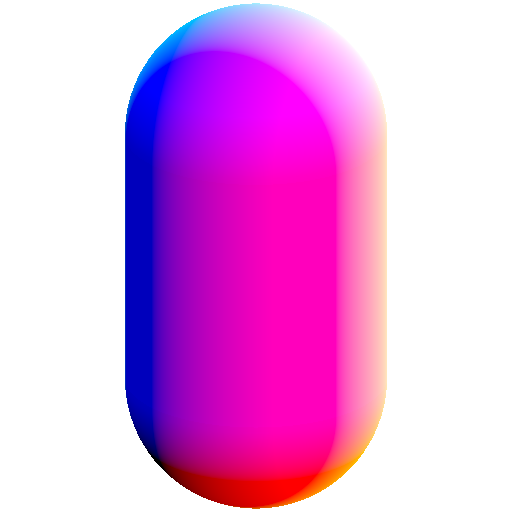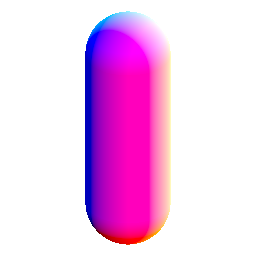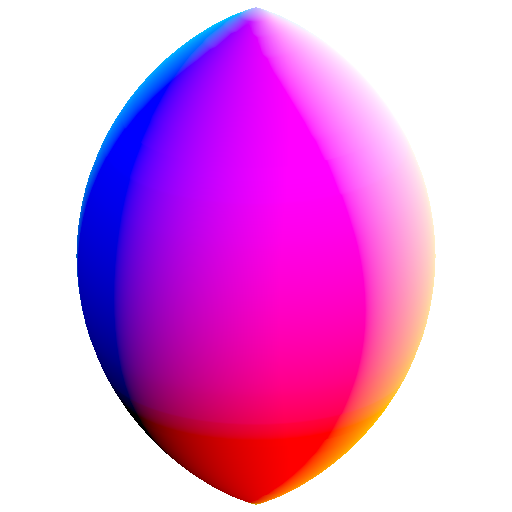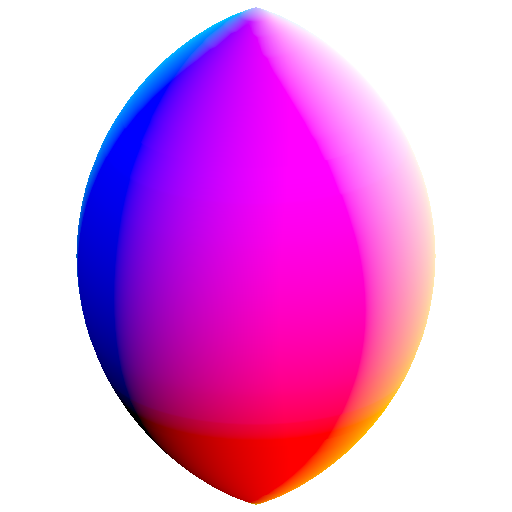for a capsule with radius 1 and cylinder height 2a, a parametric 2D equation is

(              1,           a (2t - 1)) for 0 ≤ t < 1 

( cos((t - 1) π),   a + sin((t - 1) π)) for 1 ≤ t < 2 

(             -1,   -a (2 (t - 2) - 1)) for 2 ≤ t < 3 

(-cos((t - 3) π),  -a - sin((t - 3) π)) for 3 ≤ t ≤ 4 

for a != 1, its dual biconic has a parametric 2D equation

(       2t - 1,  (a - sqrt((2t - 1)² (a² - 1) + 1)) / (a² - 1)) for 0 ≤ t < 1 

(-(2(t-1) - 1), -(a - sqrt((2t - 3)² (a² - 1) + 1)) / (a² - 1)) for 1 ≤ t ≤ 2 

for a = 1, its dual biconic has a parametric 2D equation

(       2t - 1,    1 / 2 - (2t - 1)² / 2) for 0 ≤ t < 1 

(-(2(t-1) - 1), -(1 / 2 - (2t - 1)² / 2)) for 1 ≤ t ≤ 2 

torii/torets

torii are surfaces of revolution made from circles whose centers are offset from the center of reciprocation against the axis that the shape isn't being revolved around

their duals are then a surface of revolution of a conic that has a/its focus at the center of reciprocation

Image + Name	Description	Symmetry	Dual
Ring Torus	A torus where R>r	D∞	Hyperbolet
Horn Torus	A torus where R=r	D∞	Parabolet
Spindle Torus	A torus where R<r Always topologically self-dual, but for it to also be geometrically self-dual, the circle has to be an ellipse	D∞	Elliptical Spindle Torus
Apple	Shares part of its surface with spindle torii, but only the part that is "visible from the outside" This turns the locally convex but globally self-intersecting surface into a non-intersecting surface with two concave pole points The dual is a surface of revolution of a semiellipse (stretched semicircle)	D∞	Spindlet
Single-sheeted Hyperboloid	A hyperbolet with one of the sheets removed	D∞	Semicircular Torus?

steinmetz-like shapes

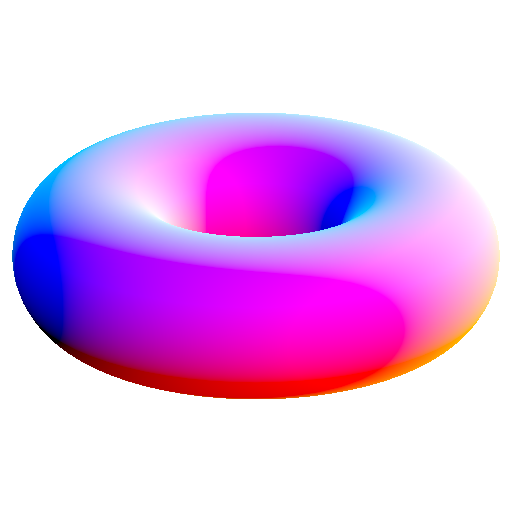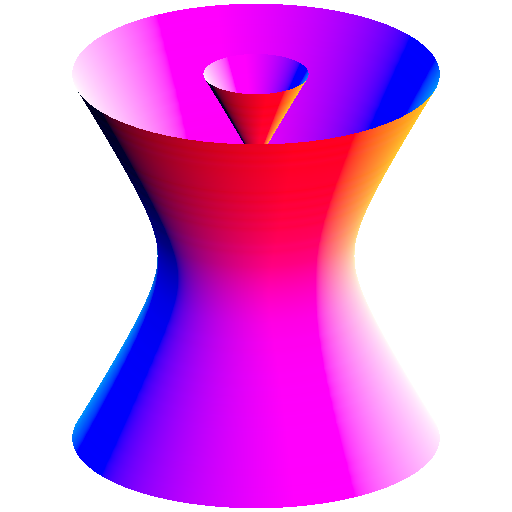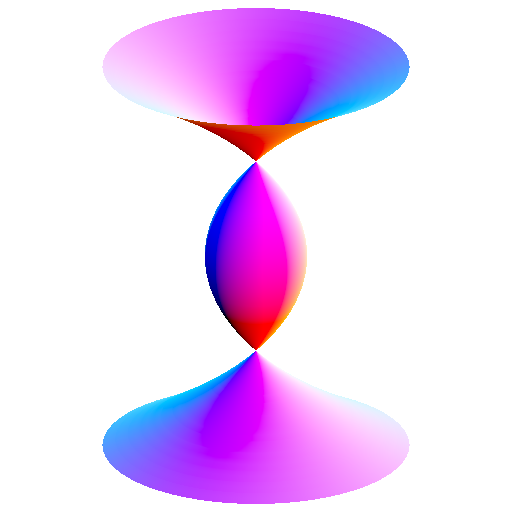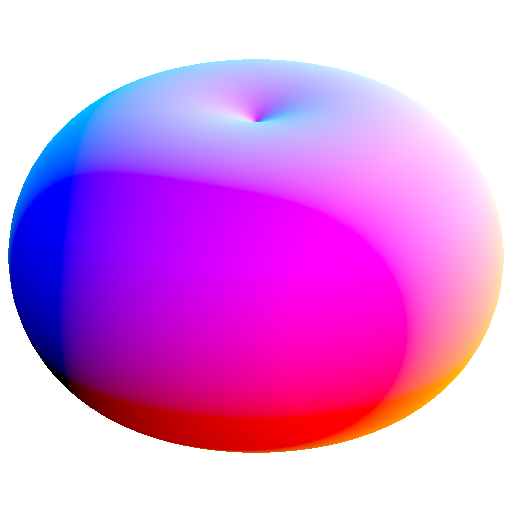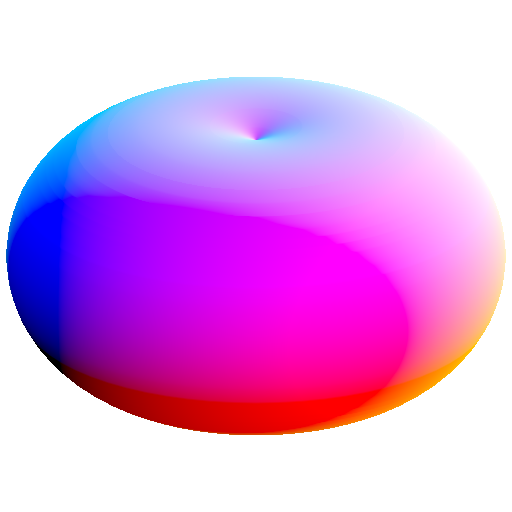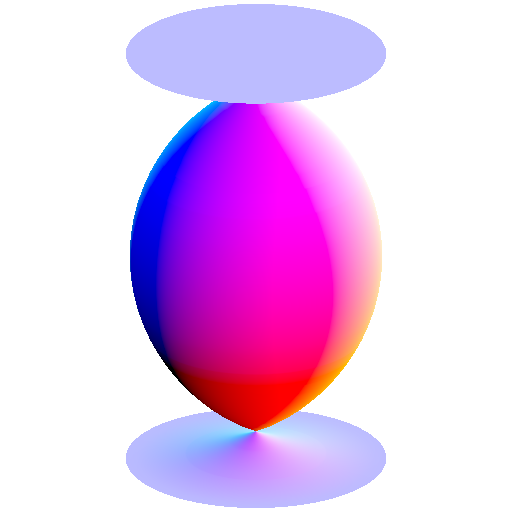Image + Name	Description	Symmetry	Dual
n-gonal Bimarengue	The convex hull of multiple semi-circles rotated around their shared center The dual is the convex core of the same amount of equivalent semi-cylinders, which happens to make it topologically self-dual	Dn	Irregular n-gonal Bimarengue
Biaxial Steinmetz Solid (Bicylinder)	The bicylinder is the convex core of two cylinders, and therefore the convex core of four semi-cylinders, making it the dual of a regular square bimarengue It has an inradius and no circumradius, and conversely the dual has a circumradius and no inradius	Dn	Regular Square Bimarengue
Triaxial Steinmetz Solid (Tricylinder)	The convex core of three cylinders whose axes are aligned with the faces of a cube The dual is the convex hull of three circles whose normal vectors are aligned with the faces of a cube	Oh	Tetratetrahedral Marenguoid
Cuboctahedral Steinmetzoid		Oh	Cuboctahedral Marenguoid
Icosidodecahedral Steinmetzoid		Ih	Icosidodecahedral Marenguoid

spheres

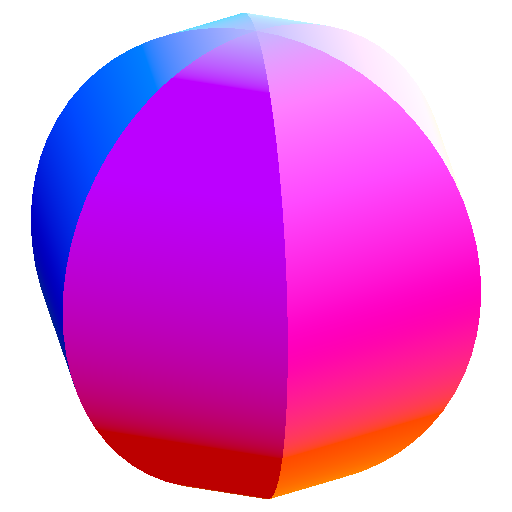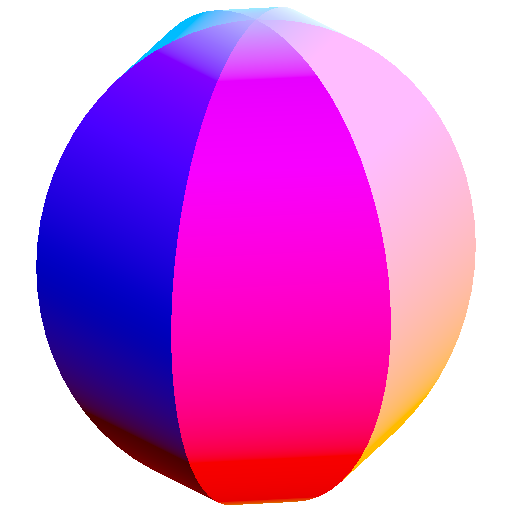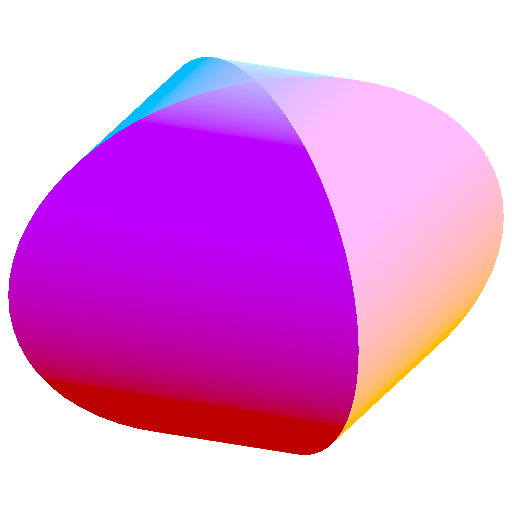
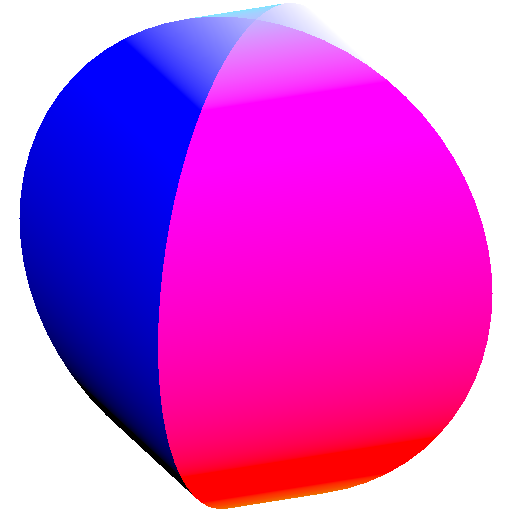
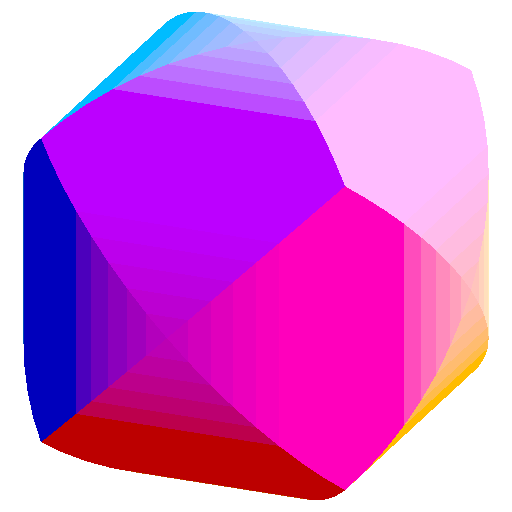
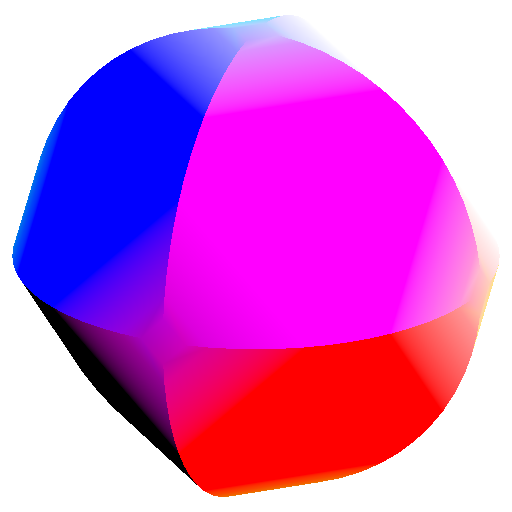
these are spheres whose center is shifted away form the center of reciprocation

because of symmetry, this can be thought of as the surfaces of revolution of a circle whose center is shifted away from the center of reciprocation along the axis of revolution

this produces duals that are a surface of revolution of a conic that has a/its focus at the center of reciprocation

Image + Name	Description	Symmetry	Dual
Sphere (Centered)		SO(3)	Sphere (Centered)
Sphere (Off-center)	Center of reciprocation is inside the sphere, but not at its center	C∞	Spheroid (Off-center)
Sphere (Boundary)	Center of reciprocation is on the boundary of the sphere	C∞	Infinite Paraboloid
Sphere (Outside)	Center of reciprocation is fully outside the sphere	C∞	Infinite Two-sheeted Hyperboloid

cones

Image + Name	Description	Symmetry	Dual
Cone	if the cross-section is an equilateral triangle and the center of reciprocation is the center of the triangle, it is self-dual	C∞	Cone
Elongated Cone		C∞	Elongated Cone
Elongated Bicone		D∞	Bifrustum

rollers

Image + Name	Description	Symmetry	Dual
Sphericon		D2d	Sphericon Dual
Sub-Oloid		D2d	Sub-Oloid Dual
Oloid		D2d	Oloid Dual
Super-Oloid		D2d	Super-Oloid Dual

cube variations

Image + Name	Description	Symmetry	Dual
Cube		Oh	some kind of round octahedron
Nube		C3v
Slube		C2v
Swube		D4h
Fube		C4v
Thrube		C2v

mabeloid variations

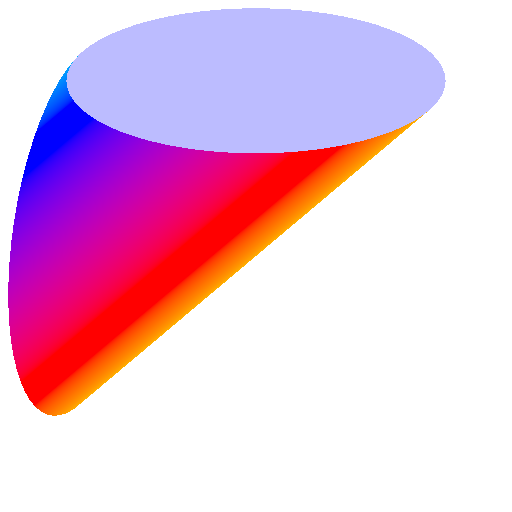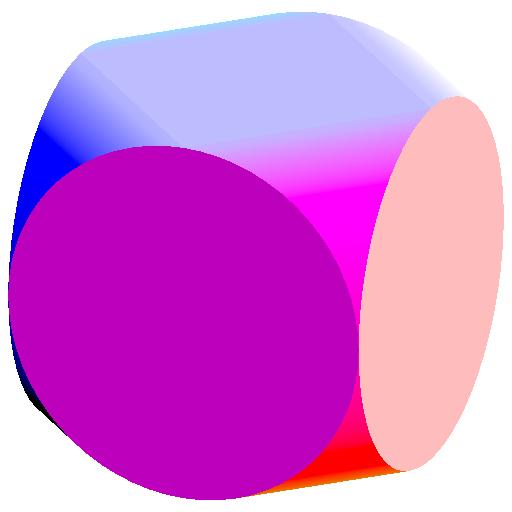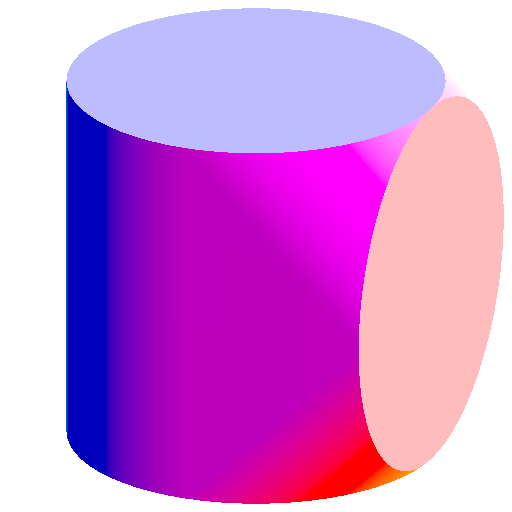the one and only

Image + Name	Description	Symmetry	Dual
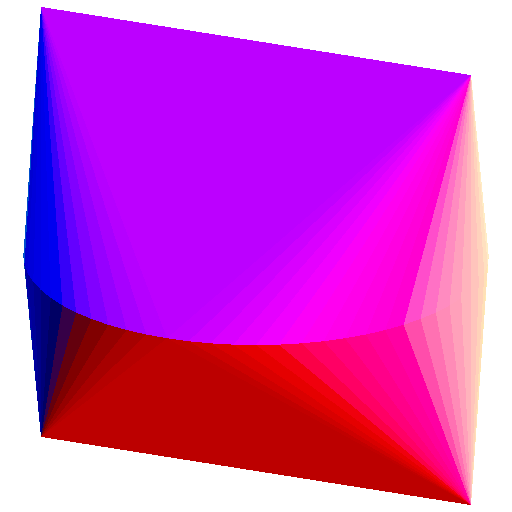Orthobimabelomabeloid		D2h	Orthobicylindromabeloid
Gyromabelocylindromabeloid	self-dual!	D2d	Gyromabelocylindromabeloid
Orthobiellipsomabeloid	i'm pretty sure this shape is discontinuous and/or non-differentiable so dualling it doesn't really converge onto a single good shape	D2h	???